Ideal Gas Law - Thermodynamics

Thermodynamics Directory | Heat Transfer Directory

Ideal Gas Law

The ideal gas law is the equation of state of a hypothetical ideal gas . It is a good approximation to the behaviour of many gases under many conditions, although it has several limitations. It was first stated by Émile Clapeyron in 1834 as a combination of Boyle's law and Charles's law .

By combining the results of Charles' and Boyle's experiments, the relationship

Pv / T = constant

may be obtained. The constant in the aboveequation is called the ideal gas constant and is designated by R; thus the ideal gas equation becomes

Pv = RT

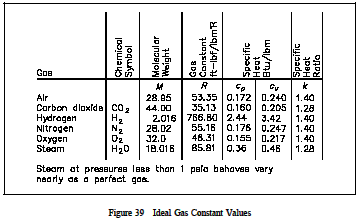
where the pressure and temperature are absolute values. The values of the ideal gas constant(R) for several of the more common gases are given in Figure 39.

The individual gas constant (R) may be obtained by dividing the universal gas constant (Ro) by the molecular weight (MW) of the gas, R = Ro / MW The units of R must always be consistentwith the units of pressure, temperature, and volume used in the gas equation. No real gases follow the ideal gas law or equation completely. At temperatures near a gases boiling point,increases in pressure will cause condensation to take place and drastic decreases in volume. At very high pressures, the intermolecular forces of a gas are significant. However, most gases are in approximate agreement at pressures and temperatures above their boiling point.

The ideal gas law is utilized by engineers working with gases because it is simple to use and approximates real gas behavior. Most physical conditions of gases used by man fit the above description. Perhaps the most common use of gas behavior studied by engineers is that of the compression process using ideal gas approximations. Such a compression process may occur at constant temperature (pV = constant), constant volume, or adiabatic (no heat transfer). Whatever the process, the amount of work that results from it depends upon the process, as brought out in the discussion on the First Law of Thermodynamics. The compression process using ideal gas considerations results in work performed on the system and is essentially the area under a P-V curve. As can be seen in Figure 40, different amounts of work result from different ideal gas processes such as constant temperature and constant pressure.