Equivalent Resistance Method - Heat Transfer

Heat Transfer Engineering
Thermodynamics

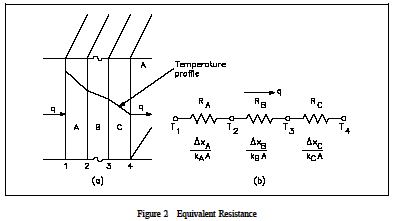
It is possible to compare heat transfer to current flow in electrical circuits. The heat transfer rate may be considered as a current flow and the combination of thermal conductivity, thickness of material, and area as a resistance to this flow. The temperature difference is the potential or driving function for the heat flow, resulting in the Fourier equation being written in a form similar to Ohms Law of Electrical Circuit Theory. If the thermal resistance term Dx/k is written as a resistance term where the resistance is the reciprocal of the thermal conductivity divided by the thickness of the material, the result is the conduction equation being analogous to electrical systems or networks.

The electrical analogy may be used to solve complex problems involving both series and parallel thermal resistance's.  The illustration given below, shows the equivalent resistance circuit. A typical conduction problem in its analogous electrical form is given in the following example, where the "electrical" Fourier equation may be written as follows.

Theory

Series Resistors

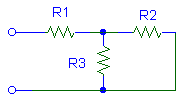
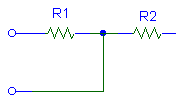
Two resistors are in series if and only if current that enters one resistor must enter the other resistor. That is, they are connected end to end in a row (but not both ends). In each of the following diagrams, R1 and R2 are in series (with respect to the terminals). Even in the last case, because no current can flow through R3.

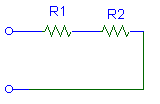
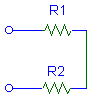
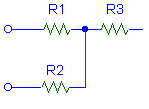
Contrast this with the following diagrams where R1 and R2 are NOT in series:

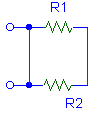
Parallel Resistors

Two resistors are in parallel if and only if the voltage is the same on both. That is, both ends are connected together. In each of the following diagrams, R1 and R2 are connected in parallel.

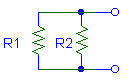
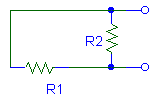
On the otherhand, in each of the diagrams below, R1 and R2 are NOT in parallel: