Related Resources: calculators
Transmission Gas Line Isothermal Flow Equations and Calculator
Fluids Engineering and Design Resources
Transmission Gas Line Isothermal Flow Equations and Calculator
The steady-state, isothermal flow behavior of gas in pipelines is defined by a general energy equation of the form:
Equation 1
Q = 38.77 · ( Tb / Pb ) · E · ( 1 / ff )0.5 · [ ( P12 - P22 ) / ( S · Lm · Tavg · Zavg ) ]0.5 · d2.5
Preview: Transmission Gas Line Isothermal Flow Calculator
This equation is completely general for steady-state flow,
and adequately accounts for variations in compressibility factor,
kinetic energy, pressure, and temperature for any typical
line section. However, the equation as derived involves an unspecified
value of the transmission factor,( 1/ff )1/2. The correct
representation of this friction factor is necessary to the validity
of the equation.
The friction factor is fundamentally related to the energy lost due to friction. In the derivation of the general energy equation, all irreversibilities and non-idealities, except for those covered by the real gas law, have been collected into the friction loss term.
Empirical methods historically and currently used to calculate or predict the flow of gas in a pipeline are the result of various correlations of the transmission factor substituted into the general energy equation.
Examination of the relationships presented by various authors shows that their forms differ primarily in the inherent or specified representation of the transmission factor which defines the energy lost in resistance to flow for various pipe sizes, roughnesses, flow conditions, and gases.
To obtain Eq 1, which is convenient for general calculations, a number of simplifying assumptions have been made. For other than pipeline sections with a very high pressure gradient, the change in the kinetic energy of the gas is not significant, and is assumed equal to zero. It is also assumed that the gas temperature is constant at an average value for the section considered; the compressibility factor is constant at the value characterized by the average gas temperature and pressure; and in the term giving the effect of elevation change, the pressure is constant at the average value. In the range of conditions to which pipeline flow equations are ordinarily applied, averages are usually sufficiently accurate. Average temperature can be cumpunter by:
Eq 2
Tavg = 0.5 ( Tin + Tout )
The average pressure in the line can be computed by:
Eq 3
Pavg = 2/ 3 [ P1 + P2 - ( P 1 P2 ) / ( P1 + P2 ) ]
In the absence of field data indicating otherwise, an efficiency factor, E, of 1.0 is usually assumed.
Where:
Q = flow rate of gas, cubic feet per day at base conditions
E = pipeline efficiency factor (fraction)
Tin = temperature in flow °R
Tout = temperature out flow °R
Tb = base absolute temperature, °R (ANSI 2530 specification:
Tb = 520°R)
Pb = base absolute pressure, psia (ANSI 2530 specification:
Pb = 14.73 psia) longitudinal weld joint factor from ANSI B31.3,
ff = Fanning friction factor
P1 = inlet pressure, psia
P2 = outlet pressure, psia
S = specific gravity of flowing gas (air = 1.0)
Lm = length of line, miles
Tavg = average temperature, °R
Pavg = average pressure, psia
Zavg = average compressibility factor
d = internal diameter of pipe, in.
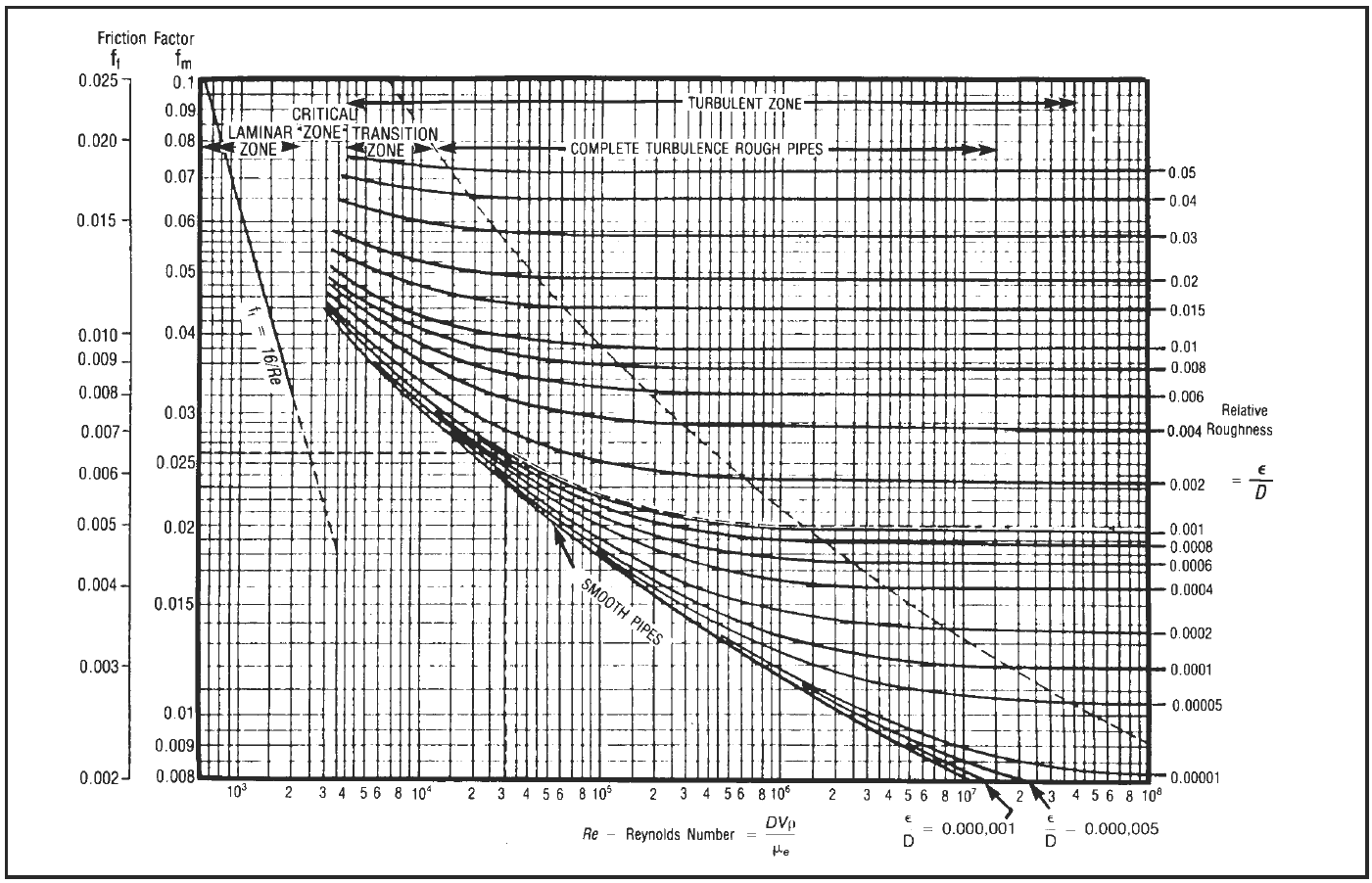
Figure 1 - Reynolds Number vs Fanning Friction Number Graph
Click on image to enlarge
Related:
- Fluids Discharge Measurement Structures and Systems , Basic Principles of Fluid Flow as Applied to Measuring Systems
- Flow of Air in Pipes Equation and Calculator
- Flow of Compressed Air in Pipes Equation and Calculator
- Flow of Water in Pipes Equations and Calculator
- Flow Rate of Steam in Schedule 40 Pipe Tables
- Pressure Drop and Flow Rate Calculations based on the Karman Method Excel Calculator Spreadsheet
- Pressure Losses for Fluid Flow in 90° Pipe Bends
- Pressure Distribution of a Fluid within a Vessel Equations and Calculator
Reference:
Gas Processors Suppliers Association, FPS Version Engineering Data Book, 2004